Fading the Gap
Introduction
To calculate the probability success when fading the opening gap in exchange traded index futures and develop a best trading strategy approach.
Assumptions and definitions
What is a gap?
For the purposes of this study it is a measure from the close of the previous trading session to the opening price of the following trading session's Regular Trading Hours (RTH). RTH is from 09:30 to 16:15 EST.
Instrument studied
This study was done on the S&P 500 e-mini contract traded electronically on CME (Symbol ES).
When has a gap filled?
It is when the a trading session's closing price has been touched during the following trading day. There is no measure of the number of days it took to fill the gap. If the gap did not fill that day then it is regarded as a "no-fill" day. On "no-fill" days the gap fade strategy closes the trade at the day's closing price at the end of RTH (in this case 16:15 EST).
It is assumed that you can enter a short/long trade at the opening price and if the gap fills/closes that you are filled at the target price even if that price is only touched and not traded beyond.
Types of Gaps
I've started to define and categorize gaps but this process isn't finished yet. For example, an Extreme Gap is when the opening price is outside the previous day's high or low and an Extreme Range Gap is when the gap is larger than the previous day's range. There are other types of gaps or sub types of gaps which are dependent on the day or the week/month or size of the gap. By categorizing and boxing these gaps I'm attempting to get a better handle on when a gap can be faded and when it shouldn't be faded.
Data Used
Daily OHLC RTH ES data from 15 January 2002 to 20 February 2004 (529 trading days with 528 gap observations).
The results of the study
The results of the study will be presented as a series of answers to questions. Charts will be presented as often as possible. The profits and losses are shown in ES points unless otherwise stated.
Limitations of study
Because the data set only has OHLC figures for the ES it is impossible to tell if the gap closed (if it did indeed close) before the most adverse movement (draw down) happened for any one day. For this reason the worst is assumed and if I use a stop loss in any of the studies it is assumed that the stop loss is hit before the gap is closed if the draw down is within the range of the stop loss.
Gap fill probability
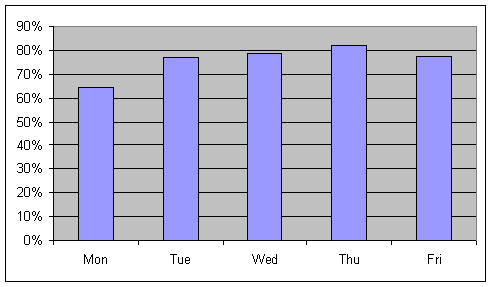
What is the probability of a gap fill (total) and which day of the week is a gap fill most likely?
| Mon | Tue | Wed | Thu | Fri | Total | |
| Gap Filled | 64 | 84 | 85 | 86 | 83 | 402 |
| Days Observed | 99 | 109 | 108 | 105 | 107 | 528 |
| Percent | 65% | 77% | 79% | 82% | 78% | 76% |
On average 76% or three quarters of all gaps close at some point during RTH.
Search this site or the web for more information on fading the opening gap:
Gap size probability
What size gaps are most likely to close?
When doing this part of the study I discovered that 14 of the days observed had no gap so the total observations in this part of the study is 514 days and not 528 as you would expect.
When looking at the tables below you should note that Gap Size is from the previous gap size + a quarter point to that gap size. So, for example, the Gap Size of 3 includes all gaps from 2.25 points to 3 points (inclusive). This gives a total of 4 possible gap sizes in each group.
| Gap Size | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| Gap Closed | 71 | 78 | 61 | 42 | 38 | 33 | 22 | 7 | 11 | 6 |
| Observations | 76 | 87 | 74 | 49 | 63 | 43 | 31 | 13 | 20 | 15 |
| Percent | 93% | 90% | 82% | 86% | 60% | 77% | 71% | 54% | 55% | 40% |
| Gap Size | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 |
| Gap Closed | 5 | 3 | 5 | 1 | 0 | 0 | 1 | 1 | 1 | 0 |
| Observations | 11 | 5 | 9 | 3 | 3 | 2 | 1 | 2 | 2 | 1 |
| Percent | 45% | 60% | 56% | 33% | 0% | 0% | 100% | 50% | 50% | 0% |
| Gap Size | 21 | 22 | 23 | 24 |
| Gap Closed | 0 | 1 | 0 | 1 |
| Observations | 0 | 1 | 1 | 2 |
| Percent | 100% | 0% | 50% |
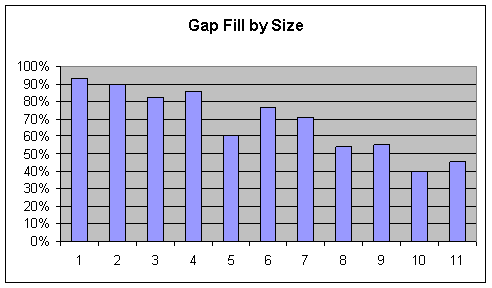
I haven't charted beyond the gap size of 11 because I felt that less that 10 observations made the results statistically insignificant.
Options Expiry Friday
Is a Gap Fill more likely on an options expiry Friday?
[NB: This calculation assumes that every third Friday of the month during the observation period was an options expiry Friday. No checks against an options expiry calendar were made to verify this.]
| All Fri | OE Fri | |
| Gap Filled | 83 | 19 |
| Days Observed | 107 | 25 |
| Percent | 78% | 76% |
The answer is NO.
Monday following Options Expiry Friday
Is a Gap Fill more likely on a Monday following an options expiry Friday?
Read NB in
| All Mon | Post OE Mon | |
| Gap Filled | 64 | 11 |
| Days Observed | 99 | 21 |
| Percent | 65% | 52% |
The answer is NO.
Rollover Days
Is a Gap Fill more likely on a rollover day?
Assumption: The second Thursday of every quarter is rollover day.
| All Thursdays | Rollover Thursdays | |
|---|---|---|
| Gap Filled | 86 | 6 |
| Days Observed | 105 | 8 |
| Percent | 82% | 75% |
Thursday is the most likely day for a gap fill. On rollover Thursdays it appears that it is less likely to fill but 75% is still a high number. Eight observations is paltry though.
If, however, you had faded the gap on those 8 rollover Thursdays you would have made a total of 28 points on the 8 trades. This is 3.5 points per trade which is a respectable return.
NB: The data I'm using is the continuous contract so there is a natural gap of about 1.5 points that hasn't been taken into account in these calculations which is an effect from rolling from one contract to another. The following future usually trades at a 1.5 point discount to expiring future so the "natural" or "neutral" gap will be -1.5 ceteris paribus.
Here is a table of the Rollover Thursday gap trades sorted by gap size:
| Date | Open | Close | Gap | Abs Gap | Gap Closed | Max Possible Draw Down | Gap Profit Loss |
|---|---|---|---|---|---|---|---|
| 12/12/2003 | 107100 | 107300 | 0 | 0 | TRUE | -550 | 0 |
| 13/06/2003 | 99925 | 98850 | -25 | 25 | TRUE | -1650 | 25 |
| 14/03/2003 | 83475 | 83300 | 250 | 250 | TRUE | -625 | 250 |
| 12/09/2003 | 101125 | 101650 | -400 | 400 | TRUE | -600 | 400 |
| 13/09/2002 | 88150 | 89175 | -775 | 775 | TRUE | -475 | 775 |
| 13/12/2002 | 89275 | 88650 | -825 | 825 | FALSE | -700 | -625 |
| 08/03/2002 | 117075 | 116675 | 850 | 850 | TRUE | -550 | 850 |
| 14/06/2002 | 99825 | 100950 | -1300 | 1300 | FALSE | -1925 | 1125 |
Friday after Rollover Days
Is a Gap Fill more likely on a Friday after a rollover Thursday?
Assumption: This is the Friday after the second Thursday of every quarter.
| All Fridays | Friday after Rollover Thursdays | |
|---|---|---|
| Gap Filled | 83 | 5 |
| Days Observed | 107 | 8 |
| Percent | 78% | 63% |
| Date | Open | Close | Gap | Abs Gap | Gap Closed | Max Possible Draw Down | Gap Profit Loss |
|---|---|---|---|---|---|---|---|
| 15/09/2003 | 101675 | 101250 | 25 | 25 | TRUE | -125 | 25 |
| 11/03/2002 | 116625 | 116900 | -50 | 50 | TRUE | -450 | 50 |
| 10/06/2002 | 102725 | 103225 | -100 | 100 | TRUE | -225 | 100 |
| 09/06/2003 | 98200 | 97700 | -575 | 575 | FALSE | -975 | -500 |
| 09/09/2002 | 88875 | 90075 | -600 | 600 | TRUE | -625 | 600 |
| 10/03/2003 | 82075 | 80775 | -775 | 775 | FALSE | -1525 | -1300 |
| 09/12/2002 | 90475 | 88950 | -925 | 925 | FALSE | -1575 | -1525 |
| 15/12/2003 | 108325 | 106750 | 1025 | 1025 | TRUE | -25 | 1025 |
The gap closed a measly 5 out of those 8 observed trades with a total loss of 15.25 points or -1.91 points per trade.
First/Last day of the month
What is the probability of a gap closing on the first/last trading day of the month?
It's difficult to say whether or not 25 days of data is significant for this study but the results are shown in the table below:
| First Session of Month | Last Session of Month | |
|---|---|---|
| Gap Filled | 14 | 21 |
| Days observed | 25 | 25 |
| Percent | 56% | 84% |
Outside/Inside Day or NR7
What is the probability of the gap closing after an Outside/Inside Day or NR7?
| OD | ID | NR7 | |
|---|---|---|---|
| Gap Filled | 38 | 42 | 48 |
| Days Observed | 58 | 56 | 69 |
| Percent | 66% | 75% | 70% |
| Gross P/L | -76.50 | 44.50 | 29.25 |
| Net P/L | -83.75 | 37.50 | 20.63 |
| Net P/L per trade | -1.44 | 0.67 | 0.30 |
Net P/L assumes that each trade costs one eighth on an ES point to execute in commissions.
Inside Days, Outside Days, Narrow Range 7 etc. were made popular by Toby Crabel in his book Day Trading with Short Term Price Patterns & Opening Range Breakout .
Back test fading all gaps
What is the probability of the gap closing after an Outside/Inside Day or NR7?
| Total | Per trade/day | |
| Gross Profit | 356.50 | 0.69 |
| Commission | 64.25 | 0.13 |
| Net | 292.25 | 0.57 |
The above table shows that we would have achieved just over 2 ticks of profit on average per trade/day had we faded every gap over the 25 months (514 days with gaps) studied.
Our best day would have shown a profit of 23.50 and our worst day a loss of 24.50
This is what I call the Raw Gap Play. This is when we fade the gap and enter at the opening price and exit at the previous day's close if the gap fills or at the end of day's closing price if the gap doesn't fill. No stops are used.
What I love about this particular data set that I've used for the gap study is that it demonstrates the sheer brutality of the market for the newcomer or under funded trader who is trading their account to the limit. I didn't choose this data set - it was all the data I could lay my hands on.
In order to achieve an average of 0.50 points a day your account would have taken a real battering at the beginning of the trading period. Consider the following facts (day in this instance refers to trading session):
- On the first day you would have lost 15.50 points.
- Only on day 7 would your account be showing a profit.
- On day 13 you would have lost 23.00 points making your account balance -18.75
- On day 82 your account would show a loss of 72.25 points of which commissions counted for 10.25 points of those losses. This is the worse draw down your account would see.
- Your account would remain negative after that until day 110 when the balance would be 0 excluding commissions (of 0.125 points per trade) or -13.75 including commissions.
- On day 112 your account would finally show a net positive balance including commissions and only because your largest winning gap trade of 23.50 points happened on that day.
Let's assume the following:
-
20 trading days per month.
-
Account balance at the start $10,000
-
1 contract traded every day there is a gap no matter the size of the gap.
After 4 months your account would have dropped to $6,387.50
After 5.5 months your account would finally be back to its original level of $10,000
That's a long time to make nothing. The mechanics of fading the gap are probably one of the simplest to trade but overcoming the psychological effect of watching your account diminish by 36% and then still having the guts/confidence to continue trading the same strategy is the real challenge.
So this leads us to the questions of stops. Stops can be absolute stops in points or a percentage of the opening gap. What is the optimal stop for our sample of data? Using linear optimization software I ran this question across the data sample allowing the optimizer to vary the size of the stops (by percentage and then by absolute value) with the objective of maximizing profit. In order to make the percentage of gap size realistic I rounded up the stop to the next quarter point.
When using a percentage of the gap size to optimize the results it turns out that you start eliminating all of the small gaps which are the ones that make up the bulk of your profits. You eliminate these because the maximum drawdown during the day almost always exceeds the stops placed on these gaps and so they all get stopped out. In reality these gaps probably filled very early on at a profit but from our limited data set we can't tell that. So the optimum solution was to use such a large percentage that it was the equivalent of not using stops.
Exactly the same happened when using stops of fixed sizes.
The conclusion to the use of stops is NOT to not use stops but to rather look at other factors that can keep us out of unprofitable gap plays or develop a strategy of waiting for an improvement before entering a gap fade trade. Warning: Trading without stops is very dangerous
Monthly Returns
What is the monthly return (in points) over the study period?
We kept this table up-to-date for a number of months but we are no longer doing this. We may in the future run stats across many more years for the gap study. Check back or keep an eye on the Forum.
I thought the table was interesting and would like to see this table going back as many years as possible to see if there are any months in which the strategy is more likely to work. If I manage to get hold of this data then I'll expand the table.
| 2002 | 2003 | 2004 | 2005 | 2006 | 2007 | |
|---|---|---|---|---|---|---|
| Jan | -7.50 | 7.75 | 7.50 | 19.25 | 0.25 | |
| Feb | -48.00 | 20.50 | 18.00 | -5.00 | -13.50 | |
| Mar | 5.25 | -6.50 | -40.00 | 9.00 | 31.00 | |
| Apr | 7.75 | 36.25 | 15.50 | -7.75 | 11.25 | |
| May | -8.75 | 10.25 | 26.50 | 6.00 | -32.25 | |
| Jun | 71.50 | 33.00 | 0.75 | 31.00 | -30.25 | |
| Jul | 58.75 | 15.75 | 24.50 | 31.50 | -42.75 | |
| Aug | 73.25 | 34.75 | -2.25 | -18.75 | 28.25 | |
| Sep | 6.00 | -9.00 | -10.75 | -1.50 | -5.50 | |
| Oct | 19.75 | 27.25 | 15.75 | -29.50 | 21.25 | |
| Nov | -25.75 | 18.00 | 11.00 | 21.50 | -2.00 | |
| Dec | -11.00 | 11.25 | 13.00 | 15.00 | 5.00 |
Fading large gaps
Are the large gaps worth fading or are they just too risky?
There were 12 gaps during the 25 months of data observed that were larger than 15 points. Let's see what would have happened if you'd faded those 12 gaps:
| Date | Gap | Gap Closed | Max Possible Draw Down | Gap Profit/Loss with the following stops | |||
|---|---|---|---|---|---|---|---|
| None | 0.50 | -5.25 | -9.00 | ||||
| 08 May 2002 | 19.50 | FALSE | -23.00 | -19.00 | -0.50 | -5.25 | -9.00 |
| 14 May 2002 | 17.25 | FALSE | -7.00 | -5.50 | -0.50 | -5.25 | -5.50 |
| 07 Jun 2002 | -16.50 | TRUE | -0.75 | 16.50 | -0.50 | 16.50 | 16.50 |
| 26 Jun 2002 | -23.50 | TRUE | -0.50 | 23.50 | -0.50 | 23.50 | 23.50 |
| 17 Jul 2002 | 17.75 | TRUE | -8.75 | 17.75 | -0.50 | -5.25 | 17.75 |
| 24 Jul 2002 | -19.00 | TRUE | -4.00 | 19.00 | -0.50 | 19.00 | 19.00 |
| 29 Jul 2002 | 18.25 | FALSE | -29.00 | -22.50 | -0.50 | -5.25 | -9.00 |
| 15 Oct 2002 | 24.00 | FALSE | -17.75 | -17.50 | -0.50 | -5.25 | -9.00 |
| 17 Oct 2002 | 22.25 | FALSE | -3.75 | 6.25 | -0.50 | 6.25 | 6.25 |
| 24 Mar 2003 | -16.00 | FALSE | -16.75 | -13.75 | -0.50 | -5.25 | -9.00 |
| 02 Apr 2003 | 15.50 | FALSE | -12.00 | -5.00 | -0.50 | -5.25 | -9.00 |
| 07 Apr 2003 | 21.50 | TRUE | -5.00 | 21.50 | -0.50 | 21.50 | 21.50 |
| Totals | 21.25 | -6.00 | 50.00 | 54.00 | |||
Some notes about the above table
- The profit of the gap fade strategy is the position from open to close of RTH if the gap doesn't close under the no stop scenario.
- The column Max Possible Draw Down shows the most extreme adverse move that could have happened against your position during that trading day if your position was held for the entire day. It is possible that this extreme adverse move happened after the gap had closed but because there is no data available for when this move occurred and when the gap closed it is assumed that the adverse move happened before the gap closed. If this assumption is incorrect for any of the dates where the gap closed then the totals shown for the last two columns would improve the gap fade strategy.
- Even though the gap wasn't filled on the 17 October 2002 the gap fill strategy still shows a profit because the short from open to close would have shown that profit.
- Only stops for 0.25 and 0.50 points showed a loss for these 12 trades for the gap fade/fill strategy. All other stop levels (and no stops) showed profits for the total 12 trades. The two "most optimized" stop levels were shown to be 5.25 and 9.00 point stops.
Assumption
At this point of the study I'm going to throw in an assumption. As you will have noticed above I err on the side of caution and prudence and would rather show an adverse result than positive one where the data is incomplete. The assumption I make here is that each trade costs you one eighth of an ES point. The ES trades in quarter points and each quarter point is worth $12.50 and here I'm assuming that a roundtrip commission of $6.25 is what you pay per contract to trade the ES. I know that some brokers charge more and some less but this I feel is a good middle ground and you can adjust the results to show how it would have turned out using your commissions.
Conclusion
| Stop | None | 5.25 | 9.00 |
| Total Profit | 21.25 | 50.00 | 54.00 |
| Avg profit per trade | 1.77 | 4.17 | 4.50 |
| Commission per trade | 0.13 | 0.13 | 0.13 |
| Avg Net Profit per trade | 1.65 | 4.04 | 4.38 |
Stop losses with large gaps
What if I used a % of the gap size as the stop loss on the large gaps instead of a fixed stop loss?
| Date | Gap | Gap Closed | Max Possible Draw Down | Gap Profit Loss with the following stops | |||
|---|---|---|---|---|---|---|---|
| None | -25% | -50% | -100% | ||||
| 08 May 2002 | 19.50 | FALSE | -23.00 | -19.00 | -4.88 | -9.75 | -19.50 |
| 14 May 2002 | 17.25 | FALSE | -7.00 | -5.50 | -4.31 | -5.50 | -5.50 |
| 07 Jun 2002 | -16.50 | TRUE | -0.75 | 16.50 | 16.50 | 16.50 | 16.50 |
| 26 Jun 2002 | -23.50 | TRUE | -0.50 | 23.50 | 23.50 | 23.50 | 23.50 |
| 17 Jul 2002 | 17.75 | TRUE | -8.75 | 17.75 | -4.44 | 17.75 | 17.75 |
| 24 Jul 2002 | -19.00 | TRUE | -4.00 | 19.00 | 19.00 | 19.00 | 19.00 |
| 29 Jul 2002 | 18.25 | FALSE | -29.00 | -22.50 | -4.56 | -9.13 | -18.25 |
| 15 Oct 2002 | 24.00 | FALSE | -17.75 | -17.50 | -6.00 | -12.00 | -17.50 |
| 17 Oct 2002 | 22.25 | FALSE | -3.75 | 6.25 | 6.25 | 6.25 | 6.25 |
| 24 Mar 2003 | -16.00 | FALSE | -16.75 | -13.75 | -4.00 | -8.00 | -16.00 |
| 02 Apr 2003 | 15.50 | FALSE | -12.00 | -5.00 | -3.88 | -7.75 | -5.00 |
| 07 Apr 2003 | 21.50 | TRUE | -5.00 | 21.50 | 21.50 | 21.50 | 21.50 |
| Totals | 21.25 | 54.69 | 52.38 | 22.75 | |||
| Stop | None | -25% | -50% | -100% |
| Total Profit | 21.25 | 54.69 | 52.38 | 22.75 |
| Avg profit per trade | 1.77 | 4.56 | 4.36 | 1.90 |
| Commission per trade | 0.13 | 0.13 | 0.13 | 0.13 |
| Avg Net Profit per trade | 1.65 | 4.43 | 4.24 | 1.77 |
It appears that we can improve the risk/return using a 25% of gap size as the stop loss.
(The optimized % with this data turned out to be 24% with a total profit of 55.97 points.)
Fading really large gaps
What if I traded even bigger gaps?
This is cheating but I thought I'd show it to you anyway out of interest. Instead of looking at gaps that are only over 15 points we look at gaps that are only over 16 points. This excludes 2 losers from the previous study and improves our results.
| Date | Gap | Gap Closed | Max Possible Draw Down | Gap Profit Loss with the following stops | |||
|---|---|---|---|---|---|---|---|
| None | -25% | -50% | -100% | ||||
| 08 May 2002 | 19.50 | FALSE | -23.00 | -19.00 | -4.88 | -9.75 | -19.50 |
| 14 May 2002 | 17.25 | FALSE | -7.00 | -5.50 | -4.31 | -5.50 | -5.50 |
| 07 Jun 2002 | -16.50 | TRUE | -0.75 | 16.50 | 16.50 | 16.50 | 16.50 |
| 26 Jun 2002 | -23.50 | TRUE | -0.50 | 23.50 | 23.50 | 23.50 | 23.50 |
| 17 Jul 2002 | 17.75 | TRUE | -8.75 | 17.75 | -4.44 | 17.75 | 17.75 |
| 24 Jul 2002 | -19.00 | TRUE | -4.00 | 19.00 | 19.00 | 19.00 | 19.00 |
| 29 Jul 2002 | 18.25 | FALSE | -29.00 | -22.50 | -4.56 | -9.13 | -18.25 |
| 15 Oct 2002 | 24.00 | FALSE | -17.75 | -17.50 | -6.00 | -12.00 | -17.50 |
| 17 Oct 2002 | 22.25 | FALSE | -3.75 | 6.25 | 6.25 | 6.25 | 6.25 |
| 07 Apr 2003 | 21.50 | TRUE | -5.00 | 21.50 | 21.50 | 21.50 | 21.50 |
| Totals | 40.00 | 62.56 | 68.13 | 43.75 | |||
| Stop | None | -25% | -50% | -100% |
| Total Profit | 40.00 | 62.56 | 68.13 | 43.75 |
| Avg profit per trade | 4.00 | 6.26 | 6.81 | 4.38 |
| Commission per trade | 0.13 | 0.13 | 0.13 | 0.13 |
| Avg Net Profit per trade | 3.88 | 6.13 | 6.69 | 4.25 |
The optimized stop for this set of data happens to be exactly 50%.
Medians, means and modes
What are the median, mean and mode of the gap?
I'll clear the nomenclature as I go along. In calculating the median, mean and mode of the gap the absolute value of the gap was used. For these calculations we're not interested in the direction of the gap play.
The mean (also known as the average) gap of the data studied is 4.40 if you include the 14 days when no gap occurred or 4.52 if you exclude those days.
The mode of a data set is the most common occurring item in that data set or the peak of a data distribution. The mode is 2.00 points which occurred a total of 27 times. 17 of those were positive gaps and 10 were negative gaps.
The median of a data set is the halfway point when the data set is ordered by size. In other words half of the gaps are above this value and the other half below this value. Because we have 528 gaps in the study this will be the value of the gap at position number 265 when the gaps are sorted. The median is 3.25.
The standard deviation is 3.92 points.
Distribution bell curve
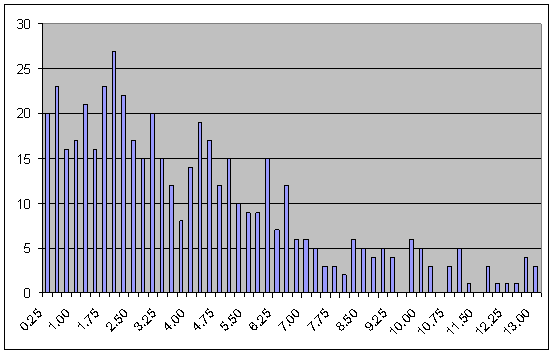
What does the distribution of the gaps look like?
This chart shows the number of times a 0.25, 0.50 etc. gap occurred in the observed data. The 14 no gap days were excluded. The eighth bar from the left shows the mode at 27 times for a gap of 2 points.

The chart below is the same chart as the one above but excludes the rare high gap occurrences for greater clarity. ( What you are looking at here is an asymmetrical bell curve in which all the values have been taken as absolute values and summed.)
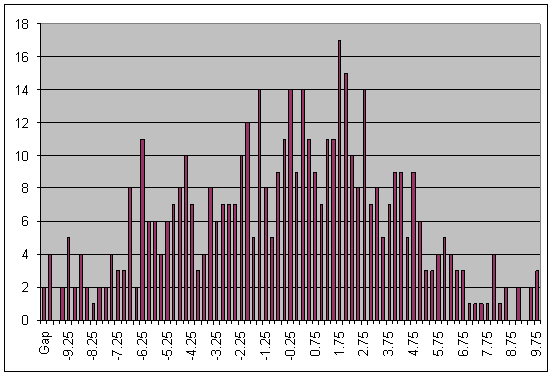
The chart below shows the number of times a gap occurred at each level from gaps of -10 to 10:
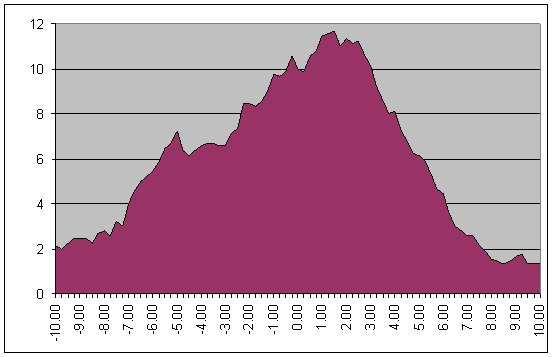
And this chart (below) is the same as the one above except that it's an area chart instead of a bar chart. I'm attempting here to create a bell curve but haven't been able to smooth out the spikes.
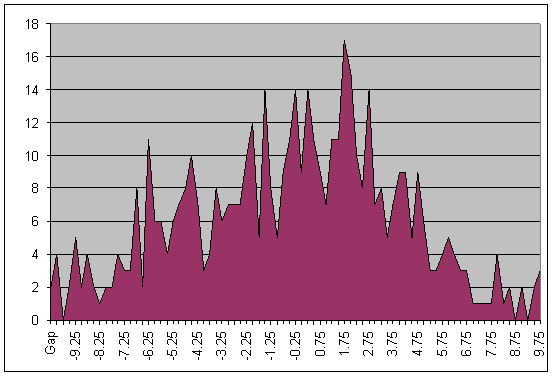
The chart below shows the average number of occurrences of a gap at each level. The average is calculated by using the number of occurrences of a gap at the level indicated on the x-axis and using the 4 values above and below that level. As such, each gap level shows the average of 9 values. I feel that this gives a good smoothed representation of the bell curve of probability of occurrences of a gap at any particular level.
This is the closest I can get to what I think is a workable and useful bell curve for the gaps on the ES. What I'm trying to produce here is not some statistically pure chart but a practical piece of information that you can print out and stick on the pin board and look at when the day starts and compare to the current day's gap.
The two pieces of information that are obvious from this chart are that the gaps are skewed to the positive side but tail off rapidly on the positive side compared to the negative side.
Gap size vs range
What is the chance of the gap filling if the gap is larger than the previous day's range?
I have called this type of gap an Extreme Range Gap. A study of the data showed that the gap was larger than the previous day's range on 17 occasions. Of those 17 times the gap filled 6 times which is only 35% of the time. If the gap fade had been traded on those 17 gaps the strategy (without stops) would have, however, return a profit of 8 points. (Commission has not been taken into account).
Here are the trades. Note how using stop loss settings of 25, 50 and 100% of the gap size yields a loss on this strategy.
| Date | Gap | Gap Closed | Max Possible Draw Down | Gap Profit Loss with the following stops | |||
|---|---|---|---|---|---|---|---|
| None | -25% | -50% | -100% | ||||
| 20 Mar 2002 | -9.75 | FALSE | -12.75 | -12.25 | -2.44 | -4.88 | -9.75 |
| 08 May 2002 | 19.50 | FALSE | -23.00 | -19.00 | -4.88 | -9.75 | -19.50 |
| 19 Jun 2002 | -15.00 | FALSE | -12.50 | -9.25 | -3.75 | -7.50 | -9.25 |
| 11 Sep 2002 | 12.75 | TRUE | -3.75 | 12.75 | -3.19 | 12.75 | 12.75 |
| 15 Oct 2002 | 24.00 | FALSE | -17.75 | -17.50 | -6.00 | -12.00 | -17.50 |
| 17 Oct 2002 | 22.25 | FALSE | -3.75 | 6.25 | 6.25 | 6.25 | 6.25 |
| 02 Dec 2002 | 12.75 | TRUE | -6.50 | 12.75 | -3.19 | -6.38 | 12.75 |
| 04 Dec 2002 | -11.75 | TRUE | -3.25 | 11.75 | -2.94 | 11.75 | 11.75 |
| 04 Feb 2003 | -9.75 | TRUE | -10.50 | 9.75 | -2.44 | -4.88 | -9.75 |
| 31 Mar 2003 | -13.00 | FALSE | -10.00 | -3.00 | -3.25 | -6.50 | -3.00 |
| 02 Apr 2003 | 15.50 | FALSE | -12.00 | -5.00 | -3.88 | -7.75 | -5.00 |
| 07 Apr 2003 | 21.50 | TRUE | -5.00 | 21.50 | 21.50 | 21.50 | 21.50 |
| 22 Sep 2003 | -9.00 | FALSE | -8.25 | -2.75 | -2.25 | -4.50 | -2.75 |
| 03 Oct 2003 | 11.75 | FALSE | -6.75 | 2.75 | -2.94 | -5.88 | 2.75 |
| 22 Oct 2003 | -8.75 | FALSE | -8.25 | -4.25 | -2.19 | -4.38 | -4.25 |
| 01 Dec 2003 | 4.50 | FALSE | -8.25 | -6.75 | -1.13 | -2.25 | -4.50 |
| 15 Dec 2003 | 10.25 | TRUE | -0.25 | 10.25 | 10.25 | 10.25 | 10.25 |
| Totals | 8.00 | -6.44 | -14.13 | -7.25 | |||
In fact the optimal stop loss on these trades turned out to 30% which produced a gross profit of just over 16 points. Stop losses of 30 to 40% of the gap size all produced profits which decrease as the percent increased from 30 to 40.
If you look at the maximum possible drawdown on each of these days it becomes apparent that if you did not fade the gap and instead had traded with the gap the trade would have gone a minimum of 3.25 points (4 Dec 2002) in your favor at some point during the day except for the Saddam gap on the 15 Dec 2003.
My conclusion to this question is: It's too risky to fade the gap if the gap is greater than the previous day's range but it mightbe worth trading with the gap instead. I say mightbecause I have done no study or defined targets for trading with the gap.
Extreme Gaps
What is the chance of an Extreme Gap filling?
I'm not sure if this type of gap already has a name but for want of a better word I've defined an Extreme Gap as: A gap where the opening price is outside the previous session's high or low.
| Extreme Gap | |
| Number of days Gap Filled | 107 |
| Number of days Observed | 159 |
| Percent filled | 67% |
| Totals | |
| Total Profit | 236.25 |
| Average Profit per Trade | 1.49 |
| Max Draw Down points of any 1 trade | 29.00 |
Interesting results on this strategy. The cumulative profit column (not shown) shows that the worst our portfolio would have suffered using this strategy was on day 6 when the cumulative profit was 4.25 points. The cumulative profit was never negative for this strategy but that is obviously because the start date did not result in a loss.
I tested this strategy with a number of different stop options and discovered that stops do not work with this strategy. No matter what stop level you used you could never improve on this by using stops. This does mean, however that you have to suffer some enormous draw down days, the worst being 29 points. On the flip side the best up day was 23.50 points.
The 107 profitable days produce 683.25 points of profit for the 107 days traded. This equates to 6.39 points average per day/trade. The 52 losing days produced 447.00 points of loss which equates to an average of 8.60 points per day/trade.
This obviously begs the question: Is there anyway we can that we can reduce the losers we trade? What happened the previous day that would give us a clue? What was the environment that could have kept us out of the losers and improved performance? Do the questions never end?
Half Gap Targets
What is the probability of half the gap filling?
For this part of the study I've tried to make it more practical and I've only looked at gaps that were bigger than 2 points. This means that the smallest gap was 2.25 points in this study. Out of the 528 days observed there were 351 days where the gap was greater than 2 points. That's 66% of the days or around 3 days out of 5.
(The reason I've limited this study to gaps greater than 2 points is because the market moves so fast when it opens that it is often impractical to enter a gap fade trade for the smaller gaps.)
Have a look at the resulting table:
| Observations | % | Total Profit * | Average Profit per trade | |
|---|---|---|---|---|
| Gaps Closed | 239 | 68% | 335.25 | 1.40 |
| Gaps Half Closed | 282 | 80% | 597.75 | 2.12 |
| Total Gaps > 2.00 points | 351 |
We see from the table that although the gap only closed 68% of the time half the gap closed 80% of the time which is a more workable figure for higher gaps sizes.
* The total profit figure is misleading in this table. It does NOT show the profit for a full gap close and a half gap close as targets as you might suspect. The total profits are calculated as follows:
Gaps Closed: This is the total profit that would have been achieved if the gap had been faded for all the trades with gaps of 2.25 or more points.
Gaps Half Closed: This is total profit that would have been achieved if the full gap had been targeted but once half the gap had been achieved a stop loss was placed at break even. (This ignores initial draw downs as no stop loss is placed until half the gap has been reached.)
There are however some flawed assumptions in this:
It assumes that every gap where half the gap was filled was also stopped out so 0 profit was recorded for that trade. It's obviously possible that this trade closed with some profit.
It also assumes that if the gap closed then the full profit of the gap was achieved. It's obviously possible that the stop was placed and hit before the profit was achieved and in this case the profit is greater than it would be in reality.
The data set, unfortunately, prevents us from seeing if the stops, targets, half-targets, extremes were hit.
Pivot Points and Gaps
Gaps and the Pivot Point and Support and Resistance Levels
| Price Opens | Seg | Closed | Total | % Closed |
|---|---|---|---|---|
| Over R3 | 7 | 1 | 1 | 100% |
| R2 to R3 | 6 | 4 | 12 | 33% |
| R1 to R2 | 5 | 21 | 34 | 62% |
| PP to R1 | 4 | 188 | 229 | 82% |
| S1 to PP | 3 | 161 | 205 | 79% |
| S2 to S1 | 2 | 21 | 37 | 57% |
| S3 to S2 | 1 | 6 | 10 | 60% |
| Below S3 | 0 | 0 | 0 | |
| Totals | 402 | 528 | 76% |
The table above shows where the ES opened in relation to the pivots for that day. I've divided the price areas into 8 segments. 6 of those segments fall between the S1, S2, S3, PP, R1, R2, and R3 lines and the other 2 segments are all the area above and below R3 and S3.
The table shows the probability of the gap closing depending on which segment the price opens in. As would be expected the two segments on either side of the Pivot Point show a higher than average probability of the gap closing.
The fact that the gap closed when the ES opened above R3 can be ignored because there is only 1 observation at this level and this is not statistically significant.
So far I have found little use in this probability table.
Now look at the tables below. I've summarized the same data but in a slightly different way to give it another perspective. The table above showed the number of times that the gap closed. If there was no gap then this was included as a gap closed. In the table below it looks at whether or not the strategy made a profit and not if the gap closed. Remember that even if the gap did not close the strategy may still have been profitable because the trade may have closed out at a profit at the end of the day. Zero gap days are not included in the table below and as such the totals show 514 trades instead of 528 days observed.
Use the first table (above) to cross reference the segments to the relevant position of the open between the support/pivot/resistance levels.
| Segment | Num Profitable | Num Not Profitable | Grand Total | % Profitable |
|---|---|---|---|---|
| 1 | 7 | 3 | 10 | 70% |
| 2 | 21 | 16 | 37 | 57% |
| 3 | 155 | 41 | 196 | 79% |
| 4 | 185 | 39 | 224 | 83% |
| 5 | 23 | 11 | 34 | 68% |
| 6 | 6 | 6 | 12 | 50% |
| 7 | 1 | 1 | 100% | |
| Grand Total | 398 | 116 | 514 | 77% |
The two tables below show the totals and averages of gap fills using the same segmentation/categorization of opening price. From the table above we can see that 82% of gaps open in segment 3 or 4. This means that 82% of the time the ES opens between S1 and R1 which in itself is no surprise. What concerns me here is the average loss per losing trade versus the average profit per winning trade.
| Segment | Total Profitable Points | Total Non Profitable Points | Grand Total |
|---|---|---|---|
| 1 | 88.50 | -10.00 | 78.50 |
| 2 | 171.50 | -140.50 | 31.00 |
| 3 | 474.25 | -423.75 | 50.50 |
| 4 | 511.50 | -376.00 | 135.50 |
| 5 | 176.25 | -116.00 | 60.25 |
| 6 | 55.50 | -76.25 | -20.75 |
| 7 | 21.50 | 21.50 | |
| Grand Total | 1499.00 | -1142.50 | 356.50 |
| Segment | Average Profitable Points | Average Non Profitable Points | Segment Average |
|---|---|---|---|
| 1 | 12.64 | -3.33 | 7.85 |
| 2 | 8.17 | -8.78 | 0.84 |
| 3 | 3.06 | -10.34 | 0.26 |
| 4 | 2.76 | -9.64 | 0.60 |
| 5 | 7.66 | -10.55 | 1.77 |
| 6 | 9.25 | -12.71 | -1.73 |
| 7 | 21.50 | 21.50 | |
| Both Average | 3.77 | -9.85 | 0.69 |
The final table in this section shows the extremes. Except for the 2 outlying segments the maximum loss always exceeded the maximum profit for any of the segments assuming that stops were not used.
| Segment | Max Loss | Max Profit |
|---|---|---|
| 1 | -4.25 | 23.50 |
| 2 | -24.50 | 19.00 |
| 3 | -23.25 | 12.50 |
| 4 | -21.50 | 13.00 |
| 5 | -22.00 | 17.75 |
| 6 | -22.50 | 12.75 |
| 7 | 21.50 | |
| Grand Total | -24.50 | 23.50 |
Opening/Closing prices
How significant is the opening price to the gap filling and the closing price for the day?
This is slightly off topic but nevertheless could be important.
Using the 514 days when there were gaps we know that 402 of those days the gap closed which is 78%.
The next question is not a gap question but an open to close question versus previous session's close: How many times did the ES close on the side that it opened? i.e. If the ES opened with a positive gap then how many times was that session's close above the previous session's close?
The answer is 315 times or 61% of the time. So just using this information we can say that on average if we have a positive gap then the ES will close above the previous session's close 61% of the time and visa versa.
Now we combine that with our gap analysis and ask the question: If the gap fills, will this session's close be on the same side as the gap? i.e. If the gap was positive and the gap closed, what percentage of the time will this session's close be above the previous session's close?
The answer is 189 times out of 402 gap closes or 47% of the time.
So although these results are marginal and do not give us any particular advantage to specific trading strategies it is nevertheless interesting that today's close is more likely to be on the same side as the opening unless the gap fills and then it is more likely (although marginally) to be on the other side.
It is also interesting to note that the probability of the gap closing is higher (78%) than the probability of previous session's close to today's close being the same sign (positive/negative) as the gap (61%).
This then raised another question in my mind. What if, instead of fading the gap to the gap fill (which is the previous session's close), we used the sign of the gap to trade from today's open to today's close. So the two questions are:
If we trade with the gap from open to close what is the total profit in points and also the number of winning trades? Also what is the result if we trade against the gap from open to close?
Of the 514 trades taken 6 of them closed at the opening price which leaves 508 trades with profits and losses (no commissions taken into account). If we faded the gap and traded from open to close it would have produced 259 winning trades (50%) for a total of 192 points profit. Trading with the gap would have produced 249 winning trades (48%) for a loss of 192 points. The missing 2% is from the 6 trades that closed even.
Copyright © 2004-2023, MyPivots. All rights reserved.